LCM of 4 and 7: Quick Calculation Guide
<!DOCTYPE html>
Finding the Least Common Multiple (LCM) of two numbers, such as 4 and 7, is a fundamental skill in mathematics. Whether you’re solving problems in school, managing schedules, or working on real-world applications, knowing how to calculate the LCM efficiently can save you time and effort. In this guide, we’ll walk you through the process step-by-step, ensuring you understand the concept and can apply it confidently. (LCM calculation, mathematical concepts, quick math tips)
What is the LCM and Why is it Important?

The Least Common Multiple (LCM) of two numbers is the smallest number that is a multiple of both. For instance, the LCM of 4 and 7 is essential in scenarios like scheduling events or solving equations. Understanding the LCM helps in simplifying fractions, finding patterns, and more. (LCM definition, importance of LCM, mathematical applications)
Step-by-Step Guide to Calculate the LCM of 4 and 7

Method 1: Listing Multiples
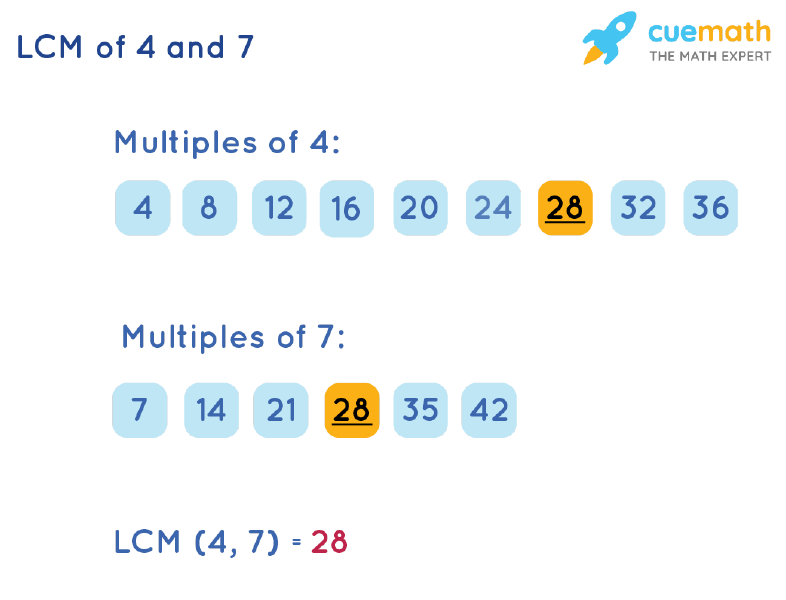
One of the simplest ways to find the LCM is by listing the multiples of each number until you find the smallest common multiple.
- Multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, …
- Multiples of 7: 7, 14, 21, 28, 35, …
The first common multiple is 28, which is the LCM of 4 and 7. (listing multiples, finding LCM, simple math methods)
Method 2: Using Prime Factorization
Another efficient method involves prime factorization. Here’s how:
- Factorize 4: 2 × 2
- Factorize 7: 7 (since 7 is a prime number)
- Multiply the highest powers of all prime factors: 2² × 7 = 4 × 7 = 28
📌 Note: Prime factorization is particularly useful for larger numbers or when dealing with more than two numbers. (prime factorization, LCM calculation methods, advanced math techniques)
LCM of 4 and 7: Quick Reference Table

| Number | Multiples |
|---|---|
| 4 | 4, 8, 12, 16, 20, 24, 28, … |
| 7 | 7, 14, 21, 28, 35, … |
| LCM | 28 |

Practical Applications of the LCM

The LCM has numerous real-world applications, including:
- Scheduling: Coordinating events or tasks that occur at different intervals.
- Cooking: Adjusting recipes to serve different numbers of people.
- Construction: Planning projects that require materials in specific quantities. (real-world LCM applications, practical math uses, everyday math)
Checklist for Calculating LCM

- Identify the numbers for which you need to find the LCM.
- Choose a method: listing multiples or prime factorization.
- Perform the calculation step-by-step.
- Verify the result by checking if it is divisible by both numbers. (LCM checklist, step-by-step LCM, math verification)
In summary, calculating the LCM of 4 and 7 is straightforward using either the listing multiples method or prime factorization. Both methods yield the same result: 28. Understanding the LCM not only helps in academic settings but also in various practical scenarios. By mastering this skill, you’ll be better equipped to tackle more complex mathematical challenges. (LCM summary, math skills, practical mathematics)
What is the LCM of 4 and 7?
+The LCM of 4 and 7 is 28.
Why is the LCM important?
+The LCM is important for simplifying fractions, scheduling, and solving real-world problems efficiently.
Can the LCM be calculated for more than two numbers?
+Yes, the LCM can be calculated for any number of values using similar methods.


