Exploring the 8gon Translation Surface: A Geometric Journey
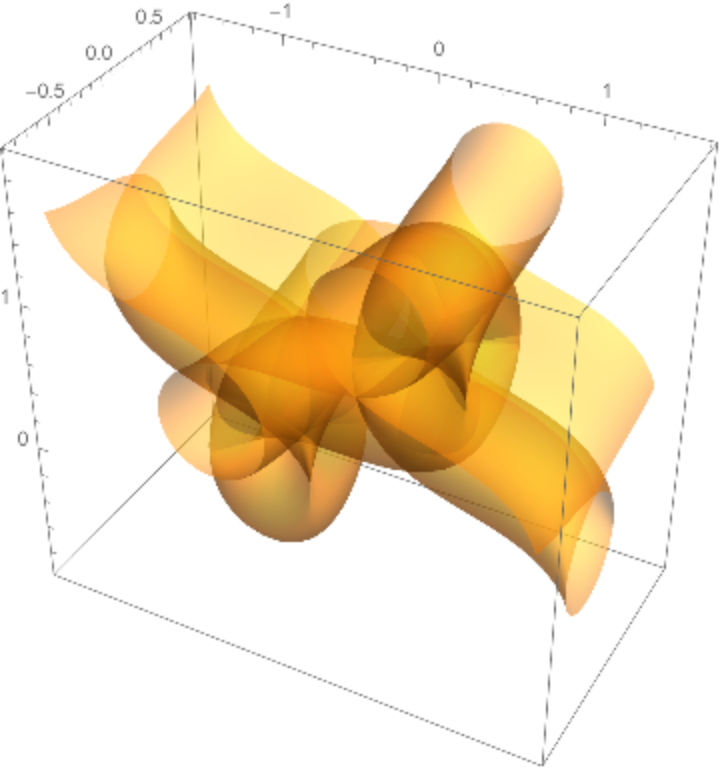
Embarking on a geometric journey through the 8gon translation surface opens up a world of mathematical beauty and complexity. This fascinating structure, rooted in geometry and topology, offers insights into symmetry, patterns, and spatial relationships. Whether you're a math enthusiast, a student, or a professional exploring applications in design and engineering, understanding the 8gon translation surface can be both enlightening and practical. Let’s dive into its intricacies, applications, and how it shapes our understanding of geometric concepts, geometric shapes, geometric patterns, and geometric design.
What is an 8gon Translation Surface?

An 8gon translation surface is a geometric structure formed by gluing together multiple regular octagons in a way that preserves their edges and vertices. This process creates a surface where translation (sliding without rotating) is the primary transformation. The result is a repeating pattern that showcases symmetry and uniformity, making it a prime example of geometric elegance.
💡 Note: The 8gon translation surface is a subset of translation surfaces, which are widely studied in mathematics for their connections to dynamics and algebra.
Key Features of the 8gon Translation Surface

- Symmetry: The surface exhibits high-order rotational and reflective symmetry, a hallmark of regular polygons like the octagon.
- Periodicity: The repeating pattern ensures that the surface is periodic, making it useful in tiling and design applications.
- Geometric Complexity: Despite its simple components, the 8gon translation surface demonstrates intricate geometric relationships.
These features make it a valuable subject in both theoretical mathematics and practical applications, such as geometric art, architecture, and material science, geometric shapes, geometric patterns, geometric design.
Applications of the 8gon Translation Surface

Mathematical Research
In mathematics, the 8gon translation surface is studied for its properties in topology and dynamical systems. Researchers use it to explore concepts like geodesic flows and billiards, geometric shapes, geometric patterns.
Design and Architecture
The surface’s periodicity and symmetry make it ideal for creating aesthetically pleasing patterns in architecture and interior design. It’s also used in the development of geometric art and decorative motifs, geometric design.
Engineering and Technology
In engineering, the principles of translation surfaces are applied to optimize structures and materials. For instance, the surface’s symmetry can inspire designs for load-bearing elements or efficient tiling systems, geometric shapes.
How to Explore the 8gon Translation Surface
- Start with Basics: Familiarize yourself with regular octagons and their properties.
- Visualize the Surface: Use geometric software or manual sketching to visualize how multiple octagons can be glued together.
- Analyze Symmetry: Identify and study the rotational and reflective symmetries present in the surface.
- Experiment with Transformations: Apply translations and other transformations to observe how the surface behaves.
📐 Note: Tools like GeoGebra or Mathematica can assist in creating and manipulating 8gon translation surfaces.
Checklist for Exploring the 8gon Translation Surface

- Understand the properties of a regular octagon.
- Visualize the gluing process to form the translation surface.
- Identify and analyze symmetries in the surface.
- Explore practical applications in design or mathematics.
- Use software tools for advanced exploration and experimentation.
The 8gon translation surface is a captivating geometric structure that bridges theory and practice. By understanding its properties and applications, you can unlock new perspectives in mathematics, design, and engineering. Whether you're exploring it for academic curiosity or practical use, this surface offers endless opportunities for discovery and innovation, geometric shapes, geometric patterns, geometric design.
What is an 8gon translation surface?
+
An 8gon translation surface is a geometric structure formed by gluing regular octagons in a way that preserves edges and vertices, creating a periodic and symmetric surface.
What are the practical applications of the 8gon translation surface?
+
It is used in mathematical research, geometric design, architecture, and engineering for its symmetry and periodicity.
How can I visualize an 8gon translation surface?
+
You can use geometric software like GeoGebra or manually sketch the gluing process of multiple octagons.



