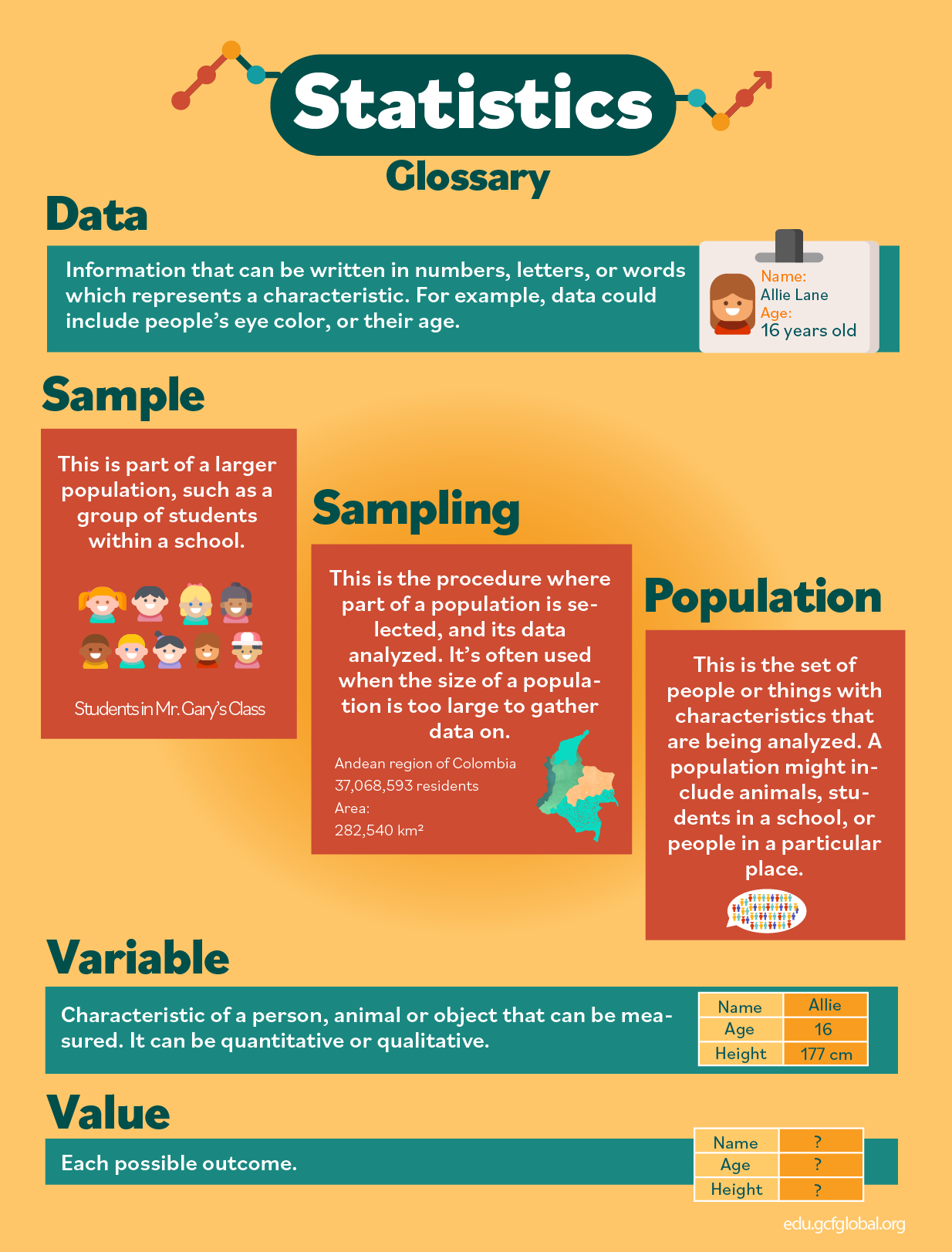
Understanding 'And' and 'Or' in Probability: Key Concepts Explained

Understanding the concepts of ‘And’ and ‘Or’ in probability is crucial for anyone looking to grasp the fundamentals of probability theory. These two logical connectors play a significant role in determining the likelihood of events occurring together or separately. In this post, we will delve into the key concepts surrounding ‘And’ and ‘Or’ in probability, providing informative copies for those seeking knowledge and commercial copies for those looking to apply these concepts in real-world scenarios (probability theory, statistical analysis, risk management).
<!DOCTYPE html>
The Basics of ‘And’ and ‘Or’ in Probability
In probability, ‘And’ represents the intersection of two events, where both events occur simultaneously. On the other hand, ‘Or’ represents the union of two events, where either one or both events occur. These concepts are essential in calculating probabilities and understanding the relationships between events (event probability, intersection, union).
Calculating Probabilities with ‘And’ and ‘Or’
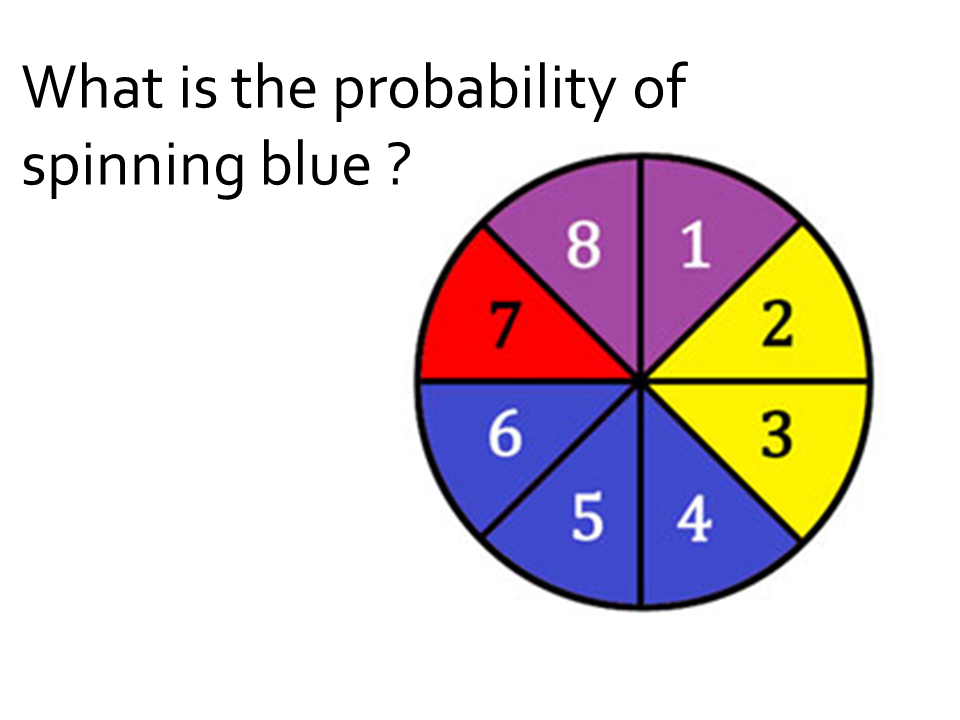
Multiplication Rule for ‘And’
When calculating the probability of two events occurring together, we use the multiplication rule: P(A and B) = P(A) × P(B | A). This rule applies when the events are dependent on each other (dependent events, conditional probability, probability calculation).
Addition Rule for ‘Or’
For calculating the probability of either one or both events occurring, we use the addition rule: P(A or B) = P(A) + P(B) - P(A and B). This rule ensures that we don’t double-count the probability of both events occurring (independent events, probability addition, event occurrence).
| Concept | Formula | Application |
|---|---|---|
| 'And' | P(A and B) = P(A) × P(B | A) | Dependent Events |
| 'Or' | P(A or B) = P(A) + P(B) - P(A and B) | Independent Events |

📝 Note: Understanding the dependence or independence of events is crucial for applying the correct formula (event dependence, independence, formula application).
Real-World Applications

The concepts of ‘And’ and ‘Or’ in probability have numerous real-world applications, including:
- Risk management in finance (financial risk, investment strategy, portfolio management)
- Quality control in manufacturing (quality assurance, defect analysis, process improvement)
- Medical diagnosis and treatment (medical probability, diagnosis accuracy, treatment effectiveness)
By mastering these concepts, you can make informed decisions and develop effective strategies in various fields (decision-making, strategy development, field-specific applications).
Checklist for Understanding ‘And’ and ‘Or’

- Identify whether events are dependent or independent
- Apply the correct formula for ‘And’ or ‘Or’
- Calculate probabilities accurately
- Interpret results in the context of real-world applications
In summary, understanding 'And' and 'Or' in probability is essential for calculating probabilities, making informed decisions, and developing effective strategies. By grasping these key concepts, you can enhance your knowledge of probability theory and its applications (probability enhancement, knowledge application, theoretical understanding).
What is the difference between 'And' and 'Or' in probability?
+'And' represents the intersection of two events, while 'Or' represents the union of two events (event intersection, union, probability difference).
When do we use the multiplication rule in probability?
+We use the multiplication rule when calculating the probability of two dependent events occurring together (dependent events, multiplication rule, probability calculation).
What are some real-world applications of 'And' and 'Or' in probability?
+Real-world applications include risk management, quality control, and medical diagnosis (risk management, quality control, medical diagnosis, probability applications).
As we wrap up, remember that a solid understanding of ‘And’ and ‘Or’ in probability is a valuable asset in various fields, from finance to healthcare. By applying these concepts effectively, you can make informed decisions, develop robust strategies, and achieve success in your endeavors (informed decision-making, strategy development, success achievement, probability theory, statistical analysis).


