Understanding the Domain for ln x: A Quick Guide

Understanding the domain of a function is crucial in mathematics, especially when dealing with logarithmic functions like ln x. The natural logarithm, denoted as ln x, is a fundamental concept in calculus and various scientific fields. This guide will walk you through understanding the domain for ln x, ensuring you grasp its significance and application.
What is the Domain of a Function?

The domain of a function refers to the set of all possible input values (x) for which the function is defined. For ln x, the domain is essential because it determines where the function can be used without leading to undefined or complex results.
Domain of ln x: Key Concepts
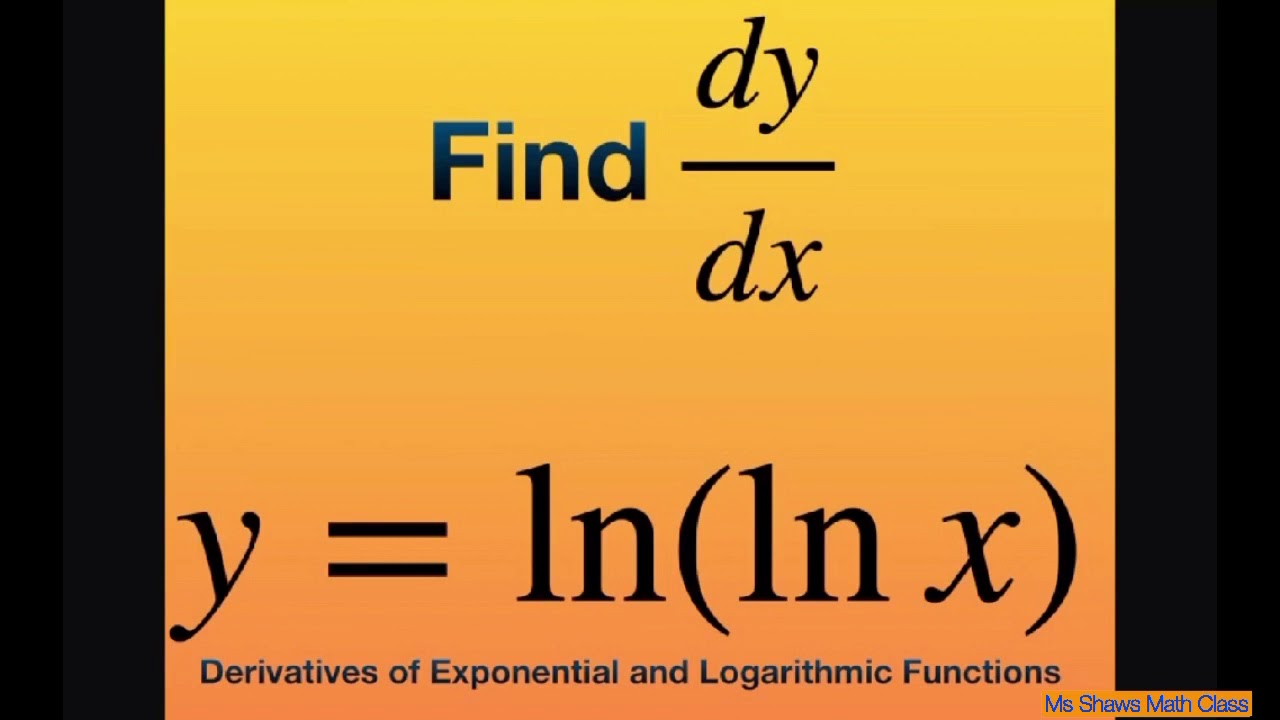
The natural logarithm function ln x is defined only for positive real numbers. This means:
- x > 0
- x cannot be zero or negative.
This restriction arises because the logarithm of zero or a negative number is undefined in the real number system.
📌 Note: The domain of ln x is strictly x > 0, excluding zero and all negative values.
Why is the Domain Important?

Understanding the domain of ln x is vital for several reasons:
1. Avoiding Errors: Applying ln x to invalid inputs (e.g., x ≤ 0) results in mathematical errors.
2. Real-World Applications: In fields like physics, biology, and economics, ln x models exponential growth, and its domain ensures realistic interpretations.
3. Mathematical Rigor: It ensures consistency and accuracy in mathematical operations.
How to Determine the Domain of ln x

To identify the domain of ln x, follow these steps:
1. Identify the Function: Confirm you’re working with ln x.
2. Apply the Rule: Recall that x > 0.
3. Exclude Invalid Values: Ensure no zero or negative values are included.
| Input (x) | Validity for ln x |
|---|---|
| x > 0 | Valid |
| x = 0 | Invalid |
| x < 0 | Invalid |

Practical Applications of ln x Domain

The domain of ln x is not just a theoretical concept; it has practical implications:
- Growth Modeling: In biology, ln x models population growth, where negative populations are nonsensical.
- Financial Calculations: In finance, ln x is used for compound interest, where negative investments are impractical.
- Scientific Research: In physics, ln x appears in equations for decay processes, where negative values are impossible.
Checklist for Working with ln x
When using ln x, keep these points in mind:
- Verify Input: Always ensure x > 0.
- Avoid Zero: Never input x = 0.
- Check Context: Ensure the domain aligns with the problem’s real-world context.
What is the domain of ln x?
+The domain of ln x is all real numbers greater than 0 (x > 0).
Why is ln x undefined for x ≤ 0?
+Ln x is undefined for x ≤ 0 because the natural logarithm of zero or negative numbers is not defined in the real number system.
How does the domain of ln x apply in real life?
+The domain ensures realistic applications in fields like biology, finance, and physics, where negative values are impractical.
In summary, the domain of ln x is a fundamental concept that ensures the function’s proper use and application. By understanding that x > 0, you can avoid errors and apply ln x effectively in both theoretical and practical scenarios. Whether you’re solving mathematical problems or modeling real-world phenomena, mastering the domain of ln x is essential for accuracy and success.
domain of ln x, natural logarithm, logarithmic functions, mathematical domain, real-world applications