How to Find the Tangent Plane: A Step-by-Step Guide
Finding the tangent plane to a surface at a given point is a fundamental concept in multivariable calculus. Whether you’re a student, researcher, or professional, understanding this process is crucial for various applications, from physics and engineering to computer graphics. In this guide, we’ll walk you through the steps to find the tangent plane, ensuring clarity and precision. Let’s dive in!
What is a Tangent Plane?

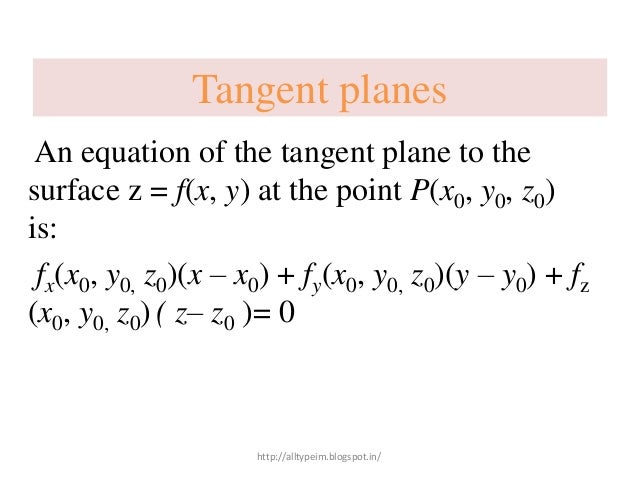
A tangent plane is a plane that touches a surface at a single point and best approximates the surface near that point. It’s essentially the multivariable analog of the tangent line in single-variable calculus. The equation of the tangent plane is derived using partial derivatives of the surface function.
Step 1: Define the Surface Function

Start by identifying the surface function ( z = f(x, y) ) or its equivalent form ( g(x, y, z) = 0 ). This function represents the surface in three-dimensional space.
📌 Note: Ensure the function is smooth and differentiable at the point of interest for accurate results.
Step 2: Compute Partial Derivatives
Next, compute the partial derivatives of the function with respect to ( x ) and ( y ). These derivatives represent the rates of change of the function in the ( x )- and ( y )-directions.
- Partial Derivative with respect to ( x ): ( f_x(x, y) )
- Partial Derivative with respect to ( y ): ( f_y(x, y) )
Step 3: Evaluate Partial Derivatives at the Point

Evaluate the partial derivatives at the specific point ( (x_0, y_0) ) where you want to find the tangent plane. These values will be used to determine the slope of the plane.
📌 Note: The point (x_0, y_0) must lie on the surface for the tangent plane to be defined.
Step 4: Write the Equation of the Tangent Plane

The equation of the tangent plane at ( (x_0, y_0, z_0) ) is given by:
[ z - z_0 = f_x(x_0, y_0)(x - x_0) + f_y(x_0, y_0)(y - y_0) ]
This equation represents the plane that best approximates the surface near the given point.
Step 5: Verify the Tangent Plane
To ensure accuracy, verify that the tangent plane touches the surface at the given point and closely approximates the surface nearby. Graphing both the surface and the plane can help visualize this relationship.
Checklist for Finding the Tangent Plane
- Identify the surface function ( z = f(x, y) ) or ( g(x, y, z) = 0 ).
- Compute partial derivatives ( f_x ) and ( f_y ).
- Evaluate derivatives at the point ( (x_0, y_0) ).
- Write the tangent plane equation using the formula above.
- Verify the plane by graphing or checking nearby points.
Why is the Tangent Plane Important?
The tangent plane is essential for linear approximation, optimization, and understanding the behavior of surfaces in multivariable calculus. It’s widely used in fields like physics, engineering, and computer graphics to model and analyze complex systems.
What is the tangent plane used for?
+The tangent plane is used for linear approximation, optimization, and understanding surface behavior in multivariable calculus.
How do partial derivatives relate to the tangent plane?
+Partial derivatives determine the slopes of the tangent plane in the x - and y -directions, defining its orientation.
Can the tangent plane exist at any point on a surface?
+The tangent plane exists only at points where the surface is smooth and differentiable.
By following these steps, you’ll be able to find the tangent plane efficiently and accurately. Whether you’re solving academic problems or applying calculus in real-world scenarios, mastering this concept is invaluable. Happy calculating! (tangent plane equation,multivariable calculus,partial derivatives)



