What is an Inverse Operation? Explained Simply
Understanding mathematical concepts can sometimes feel like unraveling a complex puzzle. One such concept that often leaves students scratching their heads is the idea of an inverse operation. But fear not! In this post, we’ll break down what an inverse operation is, why it’s important, and how it applies in both academic and real-world scenarios. Whether you’re a student, educator, or simply curious about math, this guide will simplify the concept for you. (mathematical concepts, inverse operation, real-world applications)
What is an Inverse Operation?

An inverse operation is a mathematical process that "undoes" another operation. Think of it as a reverse action. For example, if you add 5 to a number, the inverse operation would be subtracting 5 to return to the original value. Similarly, if you multiply a number by 3, the inverse operation would be dividing by 3. These pairs of operations are fundamental in algebra and problem-solving. (algebra, problem-solving, mathematical process)
Types of Inverse Operations

Addition and Subtraction
The inverse of addition is subtraction, and vice versa. For instance, if you have the equation 5 + 3 = 8, the inverse operation would be 8 - 3 = 5. This relationship is straightforward and forms the basis for more complex mathematical concepts. (addition, subtraction, mathematical concepts)
Multiplication and Division
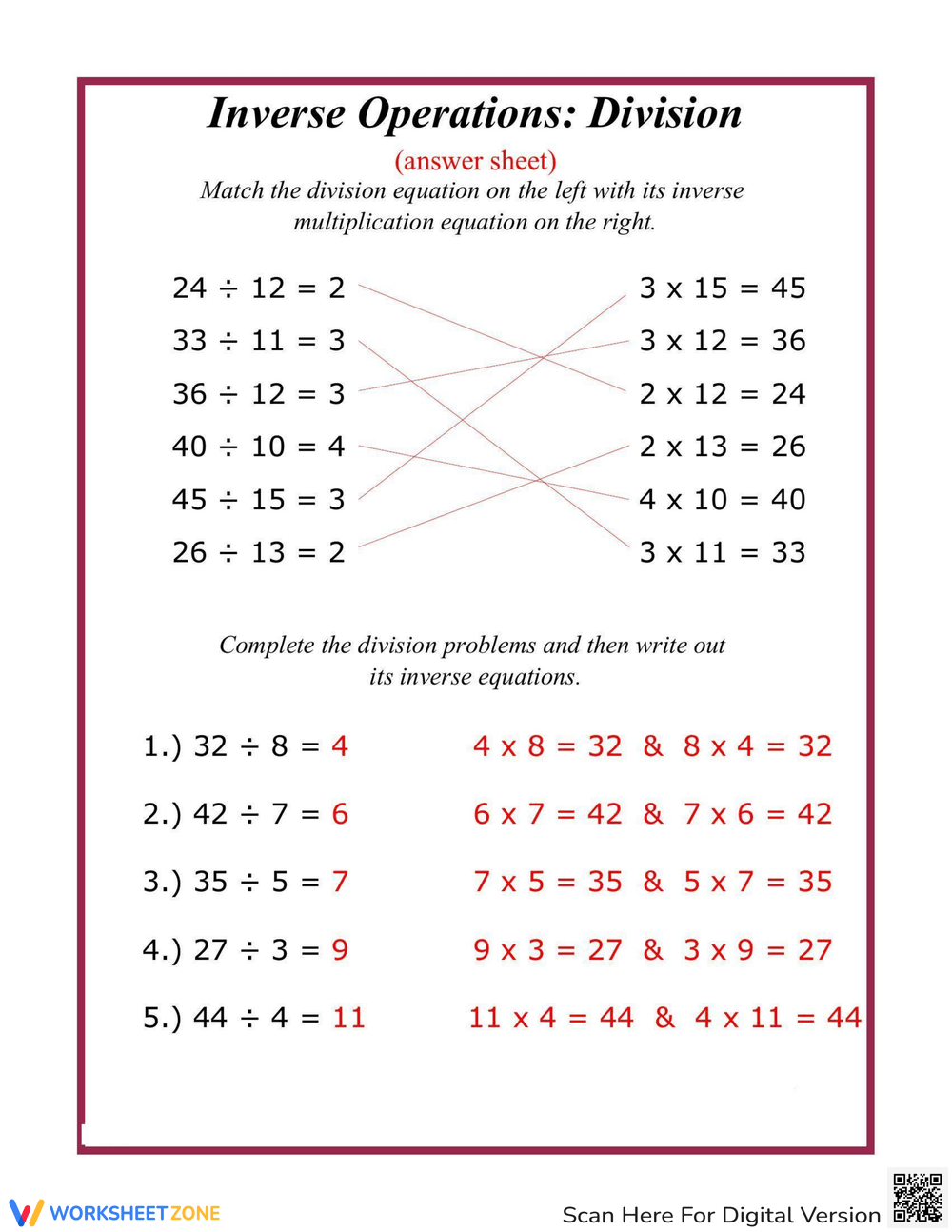
Similarly, multiplication and division are inverse operations. If you multiply 4 × 2 = 8, the inverse operation would be 8 ÷ 2 = 4. Understanding this relationship is crucial for solving equations and working with fractions. (multiplication, division, solving equations)
Exponents and Logarithms
In more advanced mathematics, the inverse of exponentiation is taking the logarithm. For example, if you have 2³ = 8, the inverse operation using logarithms would be log₂(8) = 3. This is particularly useful in fields like calculus and engineering. (exponents, logarithms, calculus)
Why Are Inverse Operations Important?

Inverse operations are essential for several reasons. They help in solving equations, checking answers, and understanding mathematical relationships. For instance, in algebra, inverse operations are used to isolate variables. In everyday life, they can help with tasks like calculating discounts or splitting bills. (solving equations, algebra, everyday life)
| Operation | Inverse Operation | Example |
|---|---|---|
| Addition | Subtraction | 5 + 3 = 8 → 8 - 3 = 5 |
| Multiplication | Division | 4 × 2 = 8 → 8 ÷ 2 = 4 |
| Exponentiation | Logarithm | 2³ = 8 → log₂(8) = 3 |

📌 Note: Inverse operations are not limited to basic arithmetic; they also apply to functions and transformations in advanced mathematics.
Summary and Checklist

To recap, inverse operations are mathematical processes that reverse each other. They are crucial for solving problems and understanding relationships in math. Here’s a quick checklist to remember:
- Addition and subtraction are inverse operations.
- Multiplication and division are inverse operations.
- Exponentiation and logarithms are inverse operations in advanced math.
- Use inverse operations to solve equations and check answers.
By mastering inverse operations, you’ll gain a stronger foundation in mathematics and improve your problem-solving skills. Whether you’re tackling homework or real-world challenges, this knowledge will serve you well. (mathematics, problem-solving skills, real-world challenges)
What is the inverse operation of multiplication?
+The inverse operation of multiplication is division. For example, if you multiply 4 by 2 to get 8, you would divide 8 by 2 to return to 4.
How are inverse operations used in algebra?
+In algebra, inverse operations are used to isolate variables in equations. For example, if you have the equation x + 5 = 10, you would subtract 5 from both sides to solve for x.
Can inverse operations be applied to functions?
+Yes, inverse operations can be applied to functions. For instance, the inverse of a linear function f(x) = 2x + 3 is f⁻¹(x) = (x - 3) / 2.